Five Ways We Undermine Efforts to Increase Student Achievement
(and what to do about it!)
by Juli Dixon
Blog Post Part 4 of 5

I am excited to provide this fourth part in a five part series of posts unpacking the Ignite session I provided at the NCTM Annual. You can view the Ignite on the NCTM Annual website (https://www.nctm.org/annual/) beginning at timestamp 21:40 but you are probably best off starting from the beginning because the other ignite sessions are excellent.
This fourth potentially (Un)Productive Practice focuses on putting the word wall in its proper place. This fourth practice is both potentially unproductive and extremely common. Teachers are expected to lead instruction with academic vocabulary. New vocabulary is introduced and defined at the start of a lesson on a new topic. The words are added to the “word wall” and referred to frequently throughout the lesson.

I just don’t understand. When we introduce new topics in mathematics instruction by starting off with defining the academic vocabulary of the lesson, we are beginning with procedures. We are saying, “here is the new word and here is what it means, now let’s make sense of it within our lesson.” How is that leading with concepts? It isn’t. This is just one reason that leading with academic vocabulary is a potentially unproductive practice.
Explore what I mean by adding the fractions below using a think aloud process before reading the text that follows the fraction problem. Feel free to write down what you do but be sure to talk through the process you use.
![]()
Did your self-talk sound something like this?

You probably didn’t need to think too much about this process. It is a procedure you know well from much practice. Now try to add the same three fractions without using the words “numerator,” “denominator,” “top number,” or “bottom number.”
This process was likely less automated. First you had to determine how to describe what you were doing to the fractions. You needed to find a way to describe what happens when you find common denominators. Your self-talk might have sounded something like this:
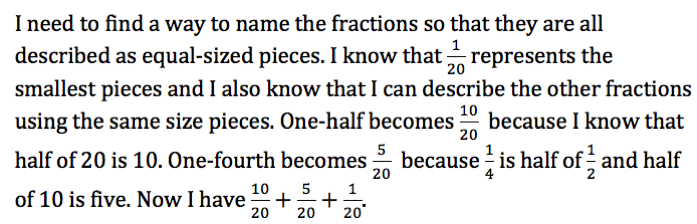
At this point you probably slipped and said that you would then add the top numbers before you realized you couldn’t say, “top numbers.” You had to pause and think about what you were doing. This is a good thing. You might have continued like this:

How does this second description compare to the first? It is more conceptual in nature. The sense making is more evident. By withholding the academic vocabulary, the process becomes more conceptual than procedural. It also becomes more accessible. We need to take a lesson from research on English language learners and lead with everyday language (Cummins, 2000). The understanding will transfer as academic language is introduced as long as the experiences are connected (I’ll talk about this more in my next blog :).
Access is another important reason for moving the placement of the word wall. If we move the word wall to the end of the lesson, more students are likely to have access to the concepts in the lesson. This is especially the case if the problems students explore are presented in context so that students have the everyday language connected to the context to use to describe the mathematics. Think about the fraction addition problem. If you had been describing pieces of cookie your language might have been more natural. I explored this with fifth-grade students in a small group setting. I describe that lesson in a book I wrote with my colleagues Lisa Brooks and Melissa Carli. The book was just recently released for pre-order through Solution Tree. The video of the lesson is included in the book using a QR code. The link to the book is included here in case you are interested: https://www.solutiontree.com/products/coming-soon/making-sense-of-math-small-groups.html.
In my work with the fifth-grade students, it was clear that the students already knew the terms numerator and denominator. In this case I disallowed the use of those terms while the focus of instruction was developing conceptual understanding of the process of adding fractions with unlike denominators. That is how I handle new concepts where the students already know the supporting vocabulary. I challenge students to describe their processes with everyday language rather than allowing students to hide behind academic vocabulary. Then, as we move towards the procedures that support the concepts we just explored, I bring the academic vocabulary back into the conversation.
Let me be clear in saying that I am not advocating for the avoidance of academic vocabulary. What I am encouraging is the practice of leading with everyday language and then naming what we learn with academic vocabulary. This mirrors leading with concepts and then after concepts are understood, providing access to more efficient procedures (although even this practice needs to be examined – it is the focus of my next post).
If you are concerned that your administrators will look for you to lead with vocabulary, please share this post with them! Have them tweet their feedback to me at @thestrokeofluck so we can all join in the conversation. We all want what’s best for our students; we just need to examine our practices to be sure that they truly make sense in the context of mathematics. Be clear that you are still going to emphasize academic vocabulary. You are just going to be more intentional about when you introduce it so that your focus is on student reasoning.
As with my other posts, the changes in practice I suggest have the goal of ensuring that the students are doing the sense making and the teacher is supporting them to meet the learning goal through the task that is chosen and the questions that are used to support the implementation of that task. As stated in the first post, we can transition our unproductive practices to be productive by keeping the learning goal and student engagement at the foreground of our planning and by critically analyzing our instructional decisions and structures. In the last post I discussed the importance of how we provide scaffolding. In this post, I added another component related to access and equity by leading with everyday language and then naming students’ new understanding with academic vocabulary. I am looking forward to reading about your reactions on twitter and continuing this conversation!
Please tweet your thoughts, comments, and ideas on this post to @thestrokeofluck
Reference:
Cummins, J. (2000). Language, power and pedagogy: Bilingual children in the crossfire.Clevedon, England: Multilingual Matters.
